Sample Solved Statistics Problems

Here we provide you with a few samples of how our work looks like.

Problem 1: A new bus route has been established between downtown Denver and Englewood, a suburb of Denver. Dan has taken the bus to work for many years. For the old bus route, he knows that the mean waiting time between buses at his stop was 18.3 minutes. However, a random sample of 5 waiting times between buses using the new route had a mean of 15.1 minutes with s = 6.2 minutes. Does this indicate that the population mean waiting time for the new route is different from what it used to be? Use = .05.

Solution: Our interest is in testing the following null and alternative hypotheses

Considering that the population standard deviation is not known, we must use a t-test with the following term:

This corresponds to a two-tailed t-test. The t-statistics is given by the following formula:

The critical value for and for degrees of freedom for this two-tailed test is. The rejection region is given by

Considering that, then we fail to reject the null hypothesis H₀.

The two-tailed p-value for this test is computed as

Since, and this leads us to the conclusion that we fail to reject the null hypothesis H₀. Therefore, we don't have enough evidence to support the claim that the population mean waiting time for the new route is different from what it used to be.

Problem 2: A company manager wishes to test a union leader’s claim that absences occur on different week days with the same frequency. To test her claim at the 0.05 significance level she collected the following sample data.

Do the data support her claim?

Solution: We are interested in testing:

We need to construct the table with observed and expected values (under the null hypothesis). The following is obtained with the information that was provided:

Using that information, we get

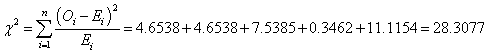
The corresponding p-value is

Since the p-value is less than the significance level, then we reject.

This means that we have enough evidence to reject the null hypothesis of equal proportions, at the 0.05 significance level.

Problem 3: You are in charge of reviewing the IQs of military personnel stationed at Rota. You know that IQ scores are normally distributed with a mean of 100 and standard deviation of 15. Base housing has 200 occupants and you have determined that results of their IQ testing mimic the population as a whole. At a briefing your colonel wants to know how many of these soldiers have an IQ of

a) At least 100

b) Between 85 and 115

c) Not more than 70

What answers do you provide the colonel?

Solution: (a) We need to compute the following probability:

so the expected number is 200*0.5 = 100 soldiers.

(b) Now, we need to compute the following probability:

so the expected number is 200*0.6827 = 136.54 137 soldiers.

c) Finally, we need to compute the following probability:

so the expected number is 200*0.0228 = 4.56 5 soldiers.

---

How it works

Simply e-mail or fax us your problems and we'll send you a free estimate in a matter of hours. There's no obligation after that. Please make sure to include

• Your deadline
• The software you need use (Excel, SPSS, etc., or any)
• Your textbook (if any)

If you accept our price, you can pay with any major credit card through a secure payment server with Paypal. Your information is safe with them. All you need to have is a credit card.    Read more

Click here to submit your payment

Prices

We have a highly efficient work system which allows us to offer unbeatable prices, starting as low as $20/hour (depending of the complexity of your request). Don't miss the opportunity to get your free estimate.

Why us?

We are trusted Paypal merchants and have handled successfully thousands of operations, for satisfied customers. Our main goal is customer satisfaction. That is why we offer a money guarantee, which means that if you are not satisfied with our solutions, we will refund you (Please read the Terms of Service for details about our guarantee).

Topics